Magnetic Field, Loop, and Faraday (21st Day)

Spring 2015

Professor Mason

May 14 Class

Magnetic Field on Wire

First thing in class, we needed to predict and draw the direction of magnetic field including the force and which direction the magnetic field is pointing at 4 location shown in the picture below. To calculate the force, we use this formula [F vector = B vector I L vector], or [F vector = (Uo I1 I2/ 2pi r) L vector], which r is the distance between two wires. Since B is perpendicular to I, we could also say that [B vector = Uo I1 I2/ 2pi r]. 

If a magnetic field on the axis of loops has a straight line wire that gives us circular magnetic field, circular wire gives us straight line magnetic field. The picture shown above also shows a B vector graph when B vector sensor is spun around the classroom (similar to wave graph); the highest point of the graph shows the north direction, while the lowest point of the graph shows the south direction. The picture attached below shows us the experiment with the wire. We were also asked if there was any force acting on the wire shown in the picture below; the answer is no, there is no force between the wires because the direction of the current is going back and forth, therefore they cancel each other out. 

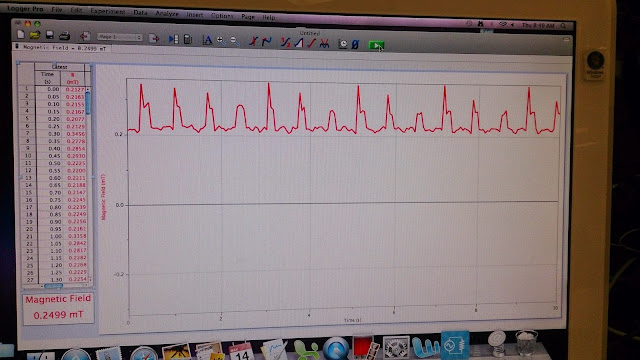
Experiment With Magnetic Field on Logger Pro.
Next, we had an experiment, which involved a power supply, test tube, copper wire, magnetic field reader, and alligator clips. The setup is shown in the picture below. In this experiment, we needed to determine how the graph of magnetic field would look like on logger pro based on how many loops encircling the test tube. We had to put the magnetic field reader close to the test tube, so that it would read accurately. 

The picture below shows the magnetic field graph of B vs T on Logger Pro with Power Supply 1 and 1 loop encircling the test tube.

 The picture below shows the magnetic field graph of B vs T on Logger Pro with Power Supply 1 and 2 loop encircling the test tube.

The picture below shows the magnetic field graph of B vs T on Logger Pro with Power Supply 1 and 3 loop encircling the test tube. 

The picture below shows the magnetic field graph of B vs T on Logger Pro with Power Supply 1 and 4 loop encircling the test tube.

The picture below shows the magnetic field graph of B vs T on Logger Pro with Power Supply 1 and 5 loop encircling the test tube. 

After we were done with power supply 1, we noticed that the big difference only lies in 1 loop and 2 loops; the rest of loops did not have much of a difference than 2 loops encircling the test tube. 

The picture below shows the magnetic field graph of B vs T on Logger Pro with Power Supply 2 and 1 loop encircling the test tube. 

The picture below shows the magnetic field graph of B vs T on Logger Pro with Power Supply 2 and 2 loop encircling the test tube. 

The picture below shows the magnetic field graph of B vs T on Logger Pro with Power Supply 2 and 3 loop encircling the test tube. 

The picture below shows the magnetic field graph of B vs T on Logger Pro with Power Supply 2 and 4 loop encircling the test tube. 

The picture below shows the magnetic field graph of B vs T on Logger Pro with Power Supply 2 and 5 loop encircling the test tube. 

When using power supply 2 (shown in the picture below), we noticed from 1 loop to 5 loops, the differences are the Magnetic Field (B); the graph increases the height as we added more loops encircling the test tube.

Galvanometer

In class, we were shown this old machine called Galvanometer as shown in the picture attached above. It is used to detect current and give an analogous reading. In this experiment, there was a  loop of wire attached up to the galvanometer, and it showed 0 A because there was no current at this point of time. The professor then took a bar of magnet and placed it into the center of the loop of wire. The galvanometer then suddenly started for a second to give a reading of some current. After he pulled it out, the galvanometer would get another reading; however, this time the galvanometer gave a negative direction. The professor then did the experiment again; however, this time he moved the bar of magnet back and forth in the center of the loop at higher speed. After that, the galvanometer received a higher reading of the magnitude of the current flowing within the wire. From this experiment, we could conclude that magnetic field can generate wireless current.

Faraday's Machine

In class, we get to see a Faraday's machine. The professor told us four things that are important in using the Faraday's Machine, and those are the strength of the magnet, the velocity of magnet's entry and exit, the radius of loop coil, and number of coils. 

The first thing he put in the Faraday's machine in this experiment was a coil with light bulb attached. Then, the bulb lights up when it is in the pole. 

The bulb did not light up again after the coil is released from the pole. 

In this experiment, the professor also provided us a copper ring, aluminum ring, steel ring, and special steel ring (has a gap in between the disk); however, we did not take pictures of them; thus we knew how they worked. First, when the professor put the copper ring inside the pole, it levitated slightly upward; next, when the professor put the aluminum ring inside the pole, it levitated higher than copper ring because aluminum is lighter than copper; next, when the professor put steel ring inside the pole, it levitated as high as copper; however, when the professor put a special steel ring in the pole, it did not levitate at all because the magnetic field was interrupted by the gap between the disk, so it did not make any circular motion. After the experiment, the professor came up with another equation, which was motion EMF [E = VB] or [E vector = V/L] or [E vector = V L B vector]. 

Falling Experiment

In class, professor did another experiment with two objects; one is magnetic and the other one is not. He also prepared two tubes; one is made of plastic glass, and the other one is made of aluminum. He purposely drop two objects at the same time through different pole. The magnetic object going through the aluminum tube fell down so much slower than the other one because the object is spinning in circular current; however, it did not stop because the gravity is pulling the object down, and it needed to move to fall down.

After the experiment, the professor came up with another equation of [E = -NA (dB/dt)] or [E = N (d(B vector dot A vector)/ dt)], which E is Lenz's Law (Induced EMF) using [Magnetic Flux = B.A]. Then, the professor gave us another equation of [B = Bo Sin wt], [Flux = pi R^2 Bo Sin wt], and [E = -Npi R^2 Bo Cos wt]. These equations are used to find the flux in a coil as function of time. Next, we also needed to draw emf vs t graph based on B vs t graph; the result looks like the graph shown in the picture attached below.